BIO 7: Lecture 28-29 Preview
Predictions made using Punnett Squares can also be made using Rules of Probability
1.
If there are m things of one kind and n things overall (where m and n
represent integers), then the probability of sampling one of those m kind of
things is:
Prob = m/n.
2.
The multiplication rule: Given two
independent events (which do not influence each other's outcome), the
probability of both events occurring is equal to the product of their individual
probabilities:
Prob (A and
B) = Prob (A) x Prob (B).
3.
The addition rule: Given two independent
events, the probability that one or the other occurs is equal to the sum of
their individual probabilities:
Prob (A or B) = Prob
(A) + Prob (B).
To apply these rules of
probability to the outcome of crosses for any single, simple trait; assume that
offspring are a a random sampling of one gene per trait from each parent. Thus:
Prob
(offspring=MM) = Prob (M from parent 1 and M from parent 2)
Prob
(offspring = mm) = Prob (m from parent 1 and m from parent 2)
Prob
(Offspring = Mm) = Prob (M from parent 1 and m from parent 2
To apply these rules of
probability to the outcome of crosses for more than one trait, remember that if
the genes for two traits assort independently, then
Prob
(specific form of trait A and specific form of trait B)
=
Mendel tested the Hypotheses of Segregation and Dominance with test crosses
Test cross: tester x other parent
recessive phenotype
homozygous recessive genotype
one kind of contribution to offspring
Expected Offspring of tester x purple F1 if hypotheses of Segregation and Dominance are correct?
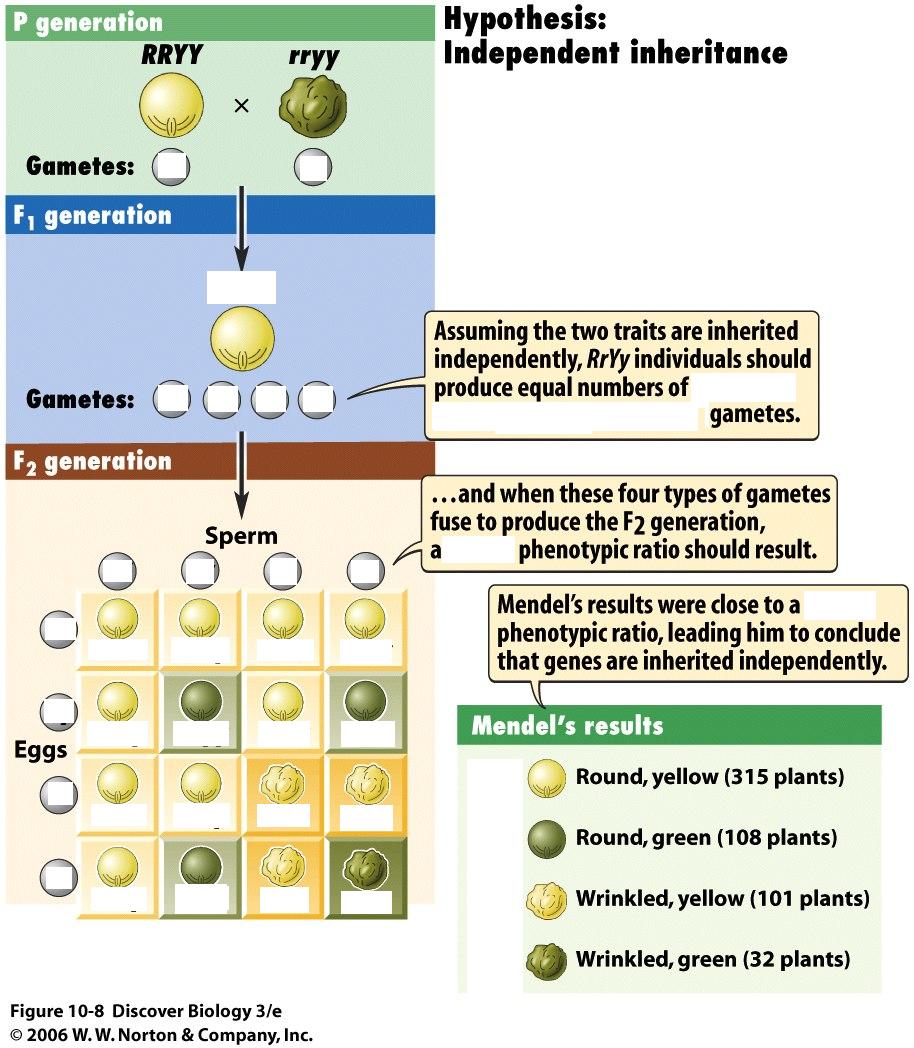
Each individual expresses many different traits. How are genes for
different traits inherited?
Mendel considered 2 extreme
hypotheses
(1)
Genes from each parent stay together; the F1 only passes on to its
offspring the combinations it received from each of its parents.
(2)
The way genes for one trait get passed on does not influence the ways
genes for a different trait get passed on;
the F1 passes on equally any combination of one gene for each trait.
Simplest case: consider 2
different traits
e.g. Trait 1 = seed color Y = yellow and y = green
Trait 2 = seed
shape R = round and r = wrinkled
How can we choose parents so
that we know the combinations of genes for seed color and shape that the F1
receives from each parent?
How can we choose a cross for
the F1 so that we know the combinations of genes the F1 passes on to its
offspring?
Prediction for outcome of
cross F1 x F1
If hypothesis 1
is correct?
If hypothesis 2
is correct?

Mendel's Observed results supported hypothesis 2, known as Mendel's Law of Independent Assortment